HSC 2015 MX2 Marathon ADVANCED (archive) (3 Viewers)

InteGrand · Jun 18, 2015

Re: HSC 2015 4U Marathon - Advanced Level

$2n<\sqrt{4n^2 + n}<2n+1$

$\left(2n+\frac{1}{4}\right)^2 = 4n^2 + n + \frac{1}{16}>4n^2 + 4n$

$$\therefore \text{frac}\left(\sqrt{4n^2 + n}\right)<\frac{1}{4}$, since the square root function is increasing.$

Sy123 · Jun 18, 2015

Re: HSC 2015 4U Marathon - Advanced Level

$\\ $Prove$ \ \frac{a}{b+c} + \frac{b}{a+c} + \frac{c}{a+b} \geq \frac{3}{2} \ $for positive$ \ a,b,c$

glittergal96 · Jun 19, 2015

Re: HSC 2015 4U Marathon - Advanced Level

Sy123 said:
$\\ $Prove$ \ \frac{a}{b+c} + \frac{b}{a+c} + \frac{c}{a+b} \geq \frac{3}{2} \ $for positive$ \ a,b,c$

Let x=a+b, y=a+c, z=b+c.

Then LHS=[(x+y-z)/z+(x+z-y)/y+(y+z-x)/x]/2=[(x/y+y/x)+(x/z+z/x)+(y/z+z/y)-3]/2 >= 3/2.

(We used AM-GM thrice in the last inequality to bound the (t+1/t) terms below by 2.)

Sy123 · Jun 19, 2015

Re: HSC 2015 4U Marathon - Advanced Level

glittergal96 said:
Let x=a+b, y=a+c, z=b+c.

Then LHS=[(x+y-z)/z+(x+z-y)/y+(y+z-x)/x]/2=[(x/y+y/x)+(x/z+z/x)+(y/z+z/y)-3]/2 >= 3/2.

(We used AM-GM thrice in the last inequality to bound the (t+1/t) terms below by 2.)

nice method, mine was a little longer:

$x = \frac{a}{a+b+c}, \ y = \frac{b}{a+b+c}, \ z = \frac{c}{a+b+c}$

$\\ \sum_{cyc} \frac{\frac{a}{a+b+c}}{1 - \frac{a}{a+b+c}} = \sum_{cyc} \frac{x}{1-x}$

$\\ f(x) = \frac{x}{1-x} \geq \frac{9}{4}x - \frac{1}{4} \\ $(found by considering the tangent to the graph at$ \ x = \frac{1}{3} \ $ then proving the inequality via calculus)$$

$\\ f(x) + f(y) + f(z) \geq \frac{9}{4} (x+y+z) - \frac{3}{4} = \frac{3}{2} \ \square$

Chlee1998 · Jun 22, 2015

Re: HSC 2015 4U Marathon - Advanced Level

suppose that a polynomial with integer coefficients satisfies P(100)=100. Find the maximum of integer solutions to P(k)=k^3 such a polynomial can have.

kawaiipotato · Jun 22, 2015

Re: HSC 2015 4U Marathon - Advanced Level

Chlee1998 said:
suppose that a polynomial with integer coefficients satisfies P(100)=100. Find the maximum of integer solutions to P(k)=k^3 such a polynomial can have.

that seems familiar to a question in the maths competition by AMT last year. couldnt do it lol

glittergal96 · Jun 23, 2015

Re: HSC 2015 4U Marathon - Advanced Level

Chlee1998 said:
suppose that a polynomial with integer coefficients satisfies P(100)=100. Find the maximum of integer solutions to P(k)=k^3 such a polynomial can have.

Can you please clarify the exact question statement?

Are these polynomials definitely required to have integer coefficients?

Is it definitely P(100)=100?

Are we meant to find the maximum number of solutions to f(k) = k^3 possible, or the largest possible k such that f(k)=k^3?

(I just want to be clear the problem is correctly written before spending time on it.)

Chlee1998 · Jun 23, 2015

Re: HSC 2015 4U Marathon - Advanced Level

glittergal96 said:
Can you please clarify the exact question statement?

Are these polynomials definitely required to have integer coefficients?

Is it definitely P(100)=100?

Are we meant to find the maximum number of solutions to f(k) = k^3 possible, or the largest possible k such that f(k)=k^3?

(I just want to be clear the problem is correctly written before spending time on it.)

a polynomial is called self centered if it has integer coefficients and p (100) = 100. if p (x) is a self centred polynomial, what is the maximum number of integer solutions to p (k) =k^3?

Drsoccerball · Jun 23, 2015

Re: HSC 2015 4U Marathon - Advanced Level

Chlee1998 said:
a polynomial is called self centered if it has integer coefficients and p (100) = 100. if p (x) is a self centred polynomial, what is the maximum number of integer solutions to p (k) =k^3?

is the answer
$k \cdot (n-2)$ ?
EDIT: n-2

Chlee1998 · Jun 23, 2015

Re: HSC 2015 4U Marathon - Advanced Level

Drsoccerball said:
is the answer
$k \cdot (n-2)$ ?
EDIT: n-2

nope, I think you've taken a wrong approach

glittergal96 · Jun 24, 2015

Re: HSC 2015 4U Marathon - Advanced Level

Okay cool, seems hard! Will spend more time on it tomorrow morning, but here is some first progress:

Since P has integer coefficients, we must have k - 100 | P(k) - P(100) = k^3 - 100.

But k^3 - 100 = (k - 100)(k^2 -100k + 10000) + 999900.

So the only k for which the identity P(k) = k^3 can possibly hold true are k such that k - 100 | 999900.

We can prime factorise this large number to obtain (2^2)(3^2)(5^2)(11)(101), which has: 2.3.3.3.2.2=216 integer factors.

So there are at most 216 integers k satisfying the property P(k) = k^3.

It is not clear however, that we can find an INTEGER polynomial passing through the points (k,k^3) for k ranging over the set {100 + d : d | 999900}, so we have only shown that 216 is an upper bound, rather than a maximum.

Will return to this tomorrow.

glittergal96 · Jun 24, 2015

Re: HSC 2015 4U Marathon - Advanced Level

Lol, woke up with an idea that I think does it.

Last post we showed that the subset S of k satisfying P(k) = k^3 consists of integers m with 100 - m a divisor of 999900.

We know that P(x) - x^3 vanishes at k in S.

So by the factor theorem we have

$P(x) = x^3 + Q(x)\prod_{k\in S} (x-k)$

for some integer polynomial Q.

Evaluating at 100, we get:

$\frac{-999900}{\prod_{k\in S} (100-k)}=Q(100)\in\mathbb{Z}.$

So we need to find a maximal set of distinct divisors of 999900 with product a factor of 999900.

By inspection, such a set is 1,-1,2,-2,3,-3,5,-5,11,101.

So: Taking Q(x)=-1 as constant and taking S={99,101,98,102,97,103,95,105,89,-1} gives us a concrete expression for an optimal P, and the answer to the question is 10.

(will double-check this in the morning, hopefully no sillies!)

simpleetal · Jun 26, 2015

Re: HSC 2015 4U Marathon - Advanced Level

here is a great question I was doing a couple of weeks back. show that in every set of 9 positive integers, a subset of 5 positive integers exists such that the sum of the numbers is divisble by 5.

SpiralFlex · Jun 26, 2015

Re: HSC 2015 4U Marathon - Advanced Level

Sy123 said:
$\\ $Prove$ \ \frac{a}{b+c} + \frac{b}{a+c} + \frac{c}{a+b} \geq \frac{3}{2} \ $for positive$ \ a,b,c$

Nesbitt's inequality is nice.

$\textup{Observe that,}$

$(\frac{a+b}{b+c} + \frac{b+c}{a+b})+ (\frac{b+c}{a+c} + \frac{a+c}{b+c})+ (\frac{c+a}{a+b} + \frac{a+b}{a+c}) \geq 2 + 2+ 2$

$\textup{Group each of the same denominators,}$

$(\frac{a+b}{b+c} + \frac{a+c}{b+c}) + (\frac{b+c}{a+b}+ \frac{c+a}{a+b})+ (\frac{b+c}{a+c} + \frac{a+b}{a+c}) \geq 6$

$\frac{2a+b+c}{b+c} + \frac{2c + a + b}{a+b} + \frac{2b + a + c}{a+c} \geq 6$

$\frac{2a}{b+c} + 1 + \frac{2c}{a+b} + 1 + \frac{2b}{a+c} + 1 \geq 6$

$\textup{The desired inequality pops out,}$

$\frac{a}{b+c} + \frac{b}{a+c} + \frac{c}{a+b} \geq \frac{3}{2}$

$\textup{Suppose that} \;x, y, z \;\textup{are positive real numbers such that}\; x + y + z = 1.$

$\textup{Prove that}\; \sqrt{6x+1}+\sqrt{6y+1} + \sqrt{6z+1} \leq 3\sqrt{3}. \;\textup{State when equality holds.}$

glittergal96 · Jun 26, 2015

Re: HSC 2015 4U Marathon - Advanced Level

SpiralFlex said:
Nesbitt's inequality is nice.

$\textup{Observe that,}$

$(\frac{a+b}{b+c} + \frac{b+c}{a+b})+ (\frac{b+c}{a+c} + \frac{a+c}{b+c})+ (\frac{c+a}{a+b} + \frac{a+b}{a+c}) \geq 2 + 2+ 2$

$\textup{Group each of the same denominators,}$

$(\frac{a+b}{b+c} + \frac{a+c}{b+c}) + (\frac{b+c}{a+b}+ \frac{c+a}{a+b})+ (\frac{b+c}{a+c} + \frac{a+b}{a+c}) \geq 6$

$\frac{2a+b+c}{b+c} + \frac{2c + a + b}{a+b} + \frac{2b + a + c}{a+c} \geq 6$

$\frac{2a}{b+c} + 1 + \frac{2c}{a+b} + 1 + \frac{2b}{a+c} + 1 \geq 6$

$\textup{The desired inequality pops out,}$

$\frac{a}{b+c} + \frac{b}{a+c} + \frac{c}{a+b} \geq \frac{3}{2}$

$\textup{Suppose that} \;x, y, z \;\textup{are positive real numbers such that}\; x + y + z = 1.$

$\textup{Prove that}\; \sqrt{6x+1}+\sqrt{6y+1} + \sqrt{6z+1} \leq 3\sqrt{3}. \;\textup{State when equality holds.}$

Cauchy-Schwartz states for real variables we have:

(ax+by+cz)^2 <= (a^2+b^2+c^2)(x^2+y^2+z^2)

with equality iff (x,y,z)=(ta,tb,tc) for some real c.

Lots of proofs of Cauchy-Schwartz are on this site, so I will assume it. (Can provide a short proof if you like, such as the one based on non-negative polynomials having non-positive discriminant).

Then C-S implies

LHS^2 =< ((6x+1)+(6y+1)+(6z+1))(1+1+1)=27.

And taking square roots of both sides finishes the question. The C-S equality condition also implies x=y=z=1/3 if we have equality.

simpleetal · Jun 26, 2015

Re: HSC 2015 4U Marathon - Advanced Level

simpleetal said:
here is a great question I was doing a couple of weeks back. show that in every set of 9 positive integers, a subset of 5 positive integers exists such that the sum of the numbers is divisble by 5.

Should I post a hint?

simpleetal · Jun 27, 2015

Re: HSC 2015 4U Marathon - Advanced Level

simpleetal said:
Should I post a hint?

Or is it the wording of the question that is throwing people off? Here is the exact wording of the question.

$\text{Given any nine integers, show that it is possible to choose five of them such that their sum is divisible by 5}$

SpiralFlex · Jun 27, 2015

Re: HSC 2015 4U Marathon - Advanced Level

glittergal96 said:
Cauchy-Schwartz states for real variables we have:

(ax+by+cz)^2 (a^2+b^2+c^2)(x^2+y^2+z^2)

with equality iff (x,y,z)=(ta,tb,tc) for some real c.

Lots of proofs of Cauchy-Schwartz are on this site, so I will assume it. (Can provide a short proof if you like, such as the one based on non-negative polynomials having non-positive discriminant).

Then C-S implies

LHS^2 =< ((6x+1)+(6y+1)+(6z+1))(1+1+1)=27.

And taking square roots of both sides finishes the question. The C-S equality condition also implies x=y=z=1/3 if we have equality.

$\textup{Or simply derive the inequality from AM-GM that is useful. Possibly one involving squares,}$

[HR][/HR]

$a^2+b^2+c^2 \geq ab+bc+ca$

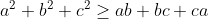
$\textup{So,}\;a^2+b^2+c^2 = (a+b+c)^2 - 2(ab+bc+ca) \geq (a+b+c)^2 - 2(a^2+b^2+c^2)$

$(a+b+c)^2 \leq 3(a^2+b^2+c^2)$

$\textup{Equality if and only if}\; a=b=c$

[HR][/HR]

$\textup{So,} \;(\sqrt{6x+1}+\sqrt{6y+1}+\sqrt{6z+1})^2 \leq 3(6x+1+6y+1+6z+1)$

$\sqrt{6x+1}+\sqrt{6y+1}+\sqrt{6z+1} \leq 3\sqrt{3}$

$\textup{Equality if and only if}\;\sqrt{6x+1}=\sqrt{6y+1}=\sqrt{6z+1}$

$\textup{Which implies}\; x=y=z = \frac{1}{3}.$

glittergal96 · Jun 27, 2015

Re: HSC 2015 4U Marathon - Advanced Level

SpiralFlex said:
$\textup{Or simply derive the inequality from AM-GM that is useful. Possibly one involving squares,}$

[HR][/HR]

$a^2+b^2+c^2 \leq ab+bc+ca$

$\textup{So.}\; (a+b+c)^2 - 2(ab+bc+ca) \leq ab+bc+ca$

$(a+b+c)^2 \leq 3(a^2+b^2+c^2)$

$\textup{Equality if and only if}\; a=b=c$

[HR][/HR]

$\textup{So,} \;(\sqrt{6x+1}+\sqrt{6y+1}+\sqrt{6z+1})^2 \leq 3(6x+1+6y+1+6z+1)$

$\sqrt{6x+1}+\sqrt{6y+1}+\sqrt{6z+1} \leq 3\sqrt{3}$

$\textup{Equality if and only if}\;\sqrt{6x+1}=\sqrt{6y+1}=\sqrt{6z+1}$

$\textup{Which implies}\; x=y=z = \frac{1}{3}.$

You have some inequalities the wrong way around, idk if your method works exactly but some tweaking might fix it.

SpiralFlex · Jun 27, 2015

Re: HSC 2015 4U Marathon - Advanced Level

glittergal96 said:
You have some inequalities the wrong way around, idk if your method works exactly but some tweaking might fix it.

Yes I will fix it give me a moment.

HSC 2015 MX2 Marathon ADVANCED (archive) (3 Viewers)

Well-Known Member

This too shall pass

Active Member

This too shall pass

Member

Well-Known Member

Active Member

Member

Well-Known Member

Member

Active Member

Active Member

Member

Well-Known Member

Active Member

Member

Member

Well-Known Member

Active Member

Well-Known Member

Users Who Are Viewing This Thread (Users: 0, Guests: 3)