Nick_K
Member
- Joined
- Mar 7, 2010
- Messages
- 64
- Gender
- Male
- HSC
- 2011
- Uni Grad
- 2014
Hi, I am stuck on a circle geometry problem and I'd appreciate any help  I'm not sure how to put a picture of the diagram up here so I'll explain it as best I can.
I'm not sure how to put a picture of the diagram up here so I'll explain it as best I can.
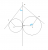
Two circles with centres H and K touch at M (They are aligned left and right, with the circle centre H on the left and K on the right). A common tangent passes through M above the circles to a point R. Another line passing through R is tangent to both circles, touching them at points P and Q (P lies on the circle with centre H, Q lies on circle with centre K).
i) Show that quadrilaterals HPRM and MRQK are cyclic. (I have done this part, just thought I'd put it in to make is clearer.)
ii) Prove that triangles PRM and MKQ are similar.
Sorry if the description isn't clear, thank you for your time.
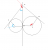
Two circles with centres H and K touch at M (They are aligned left and right, with the circle centre H on the left and K on the right). A common tangent passes through M above the circles to a point R. Another line passing through R is tangent to both circles, touching them at points P and Q (P lies on the circle with centre H, Q lies on circle with centre K).
i) Show that quadrilaterals HPRM and MRQK are cyclic. (I have done this part, just thought I'd put it in to make is clearer.)
ii) Prove that triangles PRM and MKQ are similar.
Sorry if the description isn't clear, thank you for your time.