How to find the mass and center of gravity of a bedridden patient (Physics torque question) (1 Viewer)

[Easy Peasy Lemon Squeezy]

Hi all. I have some questions about torque I can't figure out. If this is not the right place to put my question, I apologise cause it's a GAMSAT question and not a HSC question.

Sorry that this is a long post. Not allowed to distribute the exam papers so I've reworded the question slightly.

There are two similar versions of this questions. I haven't done physics so some concepts are confusing for me, but I can do the maths when I get the concept right.

Version 1:
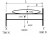
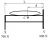
Snow White is bed-ridden. Doc wants to find her mass without moving her off the bed (see diagram). The nurse has a weighing scale that can support the pair of legs of the bed at one end or the pair at the other end, but not both pairs at the same time.
To determine the mass of the patient the nurse first places the pair of legs at X on the scale and notes a reading of 700 N. He then places the pair of legs at Y on the scale and notes a reading of 800 N.
The nurse knows that the mass of the bed and bedding is 90 kg.
With this information, he is able to find how much Snow White weighs.

Assume that the bed is stationary during weighing and the acceleration due to gravity is 10 m s–2 .

Questions:
1. The total force exerted by the floor on the bed is:
Correct Answer: 1500N
I get this, this is just 700N + 800N.

2. What is the mass of the patient?
Correct Answer: 60kg. I get this too, we know the mass of the bed is 90kg, 1500N/10 = 150, 150-90=60kg

3. Suppose the length of the bed, the horizontal distance between X and Y in Figure 1, is L.
At what horizontal distance from Y is the centre of gravity of the patient, bed and bedding, considered as a single body, located?

How do you approach this question without just guestimating? I know the working, other people have asked this online too, 700 = 1500X and I can solve for X algebraically, but I don't understand how they got to this equation.

I know torque is force x distance the force is applied at from the pivot point. 700 is the force applied at L distance from Y. But how is 1500N the force applied at x distance? The 1500N of force is spread out all along L, not at a single contact point. Even if it were at a single point, why is the center of gravity at the 7/15L mark from Y?

Since this is a torque question, and the bed is not rotating, isn't it saying 700N = 800N?

Version 2:
Same as above, but this time, you know the bed is two metres long and has an unknown mass of M.
At one end, the scales read 232kg, at the other end, it reads 220kg. The centre of mass of the patient is 0.8m from the foot of the bed. What is the mass of the patient?

Again, I know this is a question about torque but I don't see how to do it.

Thank you so much for reading.

 

[wizzkids]

Thank you for such a clear explanation of the problem, and your clear explanation of the difficulties that you are having. The good news is the answer to part (3) is quite simple, and it relies on the criterion of static equilibrium of a non-moving body.
We are going to use the theorem that the sum of all the torques or turning moments about any given point must add to Zero if a body is in static equilibrium. We are also going to use a theorem due to Newton, that the distributed mass of a body can be considered as one concentrated mass located at the centre of mass C of the body. A torque is a force multiplied by a distance.
Step 1. Pick a point, any point will do. It can be within the body or even outside the body, it doesn't matter. We use this point as our centre of rotation, or fulcrum. I suggest we choose the head of the bed, Point Y because this makes the maths easy.

Step 2. Next we identify all the external forces that are able to make the whole object rotate. These are called turning moments. The theorem of turning moments says that any force that passes straight through a point, cannot create a turning moment. Therefore the 800 N at point Y can be ignored, because it produces no turning moment. The only forces that create turning moments about point Y are the upwards 700 N force at point X and the downwards force of gravity of 1500 N at point C. The sum of these turning moments must come to zero, or else the body is not in static equilibrium.
Step 3.... can you finish the maths now?