You cannot realistically hope to learn it on BoS by today.
Locus concerns the derivation of the equation of the path of a point that moves according to given constraints, i.e. set of rules or specifications defining the path of its motion.
e.g. Find the locus of the point which is equidistant from the 2 lines:
L-1: 4x-3y+2 = 0
L-2: 3x-4y-7 = 0
Let that point be P(x,y)
^2}} \\ \\ $distance of P from line 2 is: $ d_2 = \frac {|3x-4y-7|}{\sqrt {3^2 + (-4)^2}} \\ \\ $ Now $ d_1 = d_2)
Solving, you get the equations of 2 straight lines: 1) x + y + 9 = 0 and 2) 7x - 7y - 5 = 0
These 2 lines are the bisectors of the angles between line L-1 and line l-2.
This example is relatively hard for an introduction.
A much simpler example of a locus is that of a point that is always 3 units away from a given point C. In this case the locus is a circle of radius 3. If the centre C is at the origin, this locus has the equation:
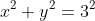
. If the centre is the point C(-5,2), then this locus is described by the equation:
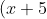^2 + (y-2)^2 = 3^2 )