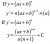intergration problem (2 Viewers)

[Fiction]

How do you solve intergration problems with something like (let f = integrand symbol) F (1-x)^4 .dx with limits of 1 and 0? Or how doul you solve integration problems which you would solve using the chain rule if it was a differentiation problem?

thanks~

 

[photastic]

Simply do reverse chain rule.
1. Add 1 to the power of the bracket.
2. Divide the bracket by the derivative of whats inside the bracket multiply the new power.

 

[turntaker]

Simply do reverse chain rule.
1. Add 1 to the power of the bracket.
2. Divide the bracket by the derivative of whats inside the bracket multiply the new power.

Why not use the differential operator.

 

[photastic]

Why not use the differential operator.

Is that even 2u?

 

[turntaker]

Is that even 2u?

No but you can use it. Its the best tool for differentiating.

Let u = 1-x
Then apply the differential operator to both sides. So,

du = -1 dx
Now we want dx by itself. So we rearrange.

dx = -du

No you can substitute back to the integral.

Int(u^5 x-du) = Int(-u^5du)
= -u^6/6 + c
= -(1-x)^6/6 + c (answer)

 

[photastic]

No but you can use it. Its the best tool for differentiating.

Let u = 1-x
Then apply the differential operator to both sides. So,

du = -1 dx
Now we want dx by itself. So we rearrange.

dx = -du

No you can substitute back to the integral.

Int(u^5 x-du) = Int(-u^5du)
= -u^6/6 + c
= -(1-x)^6/6 + c (answer)

I understand your method but this post is under mathematics. Reverse chain rule is more easier to understand and ultilise for a 2u student.

 

[HeroicPandas]

No but you can use it. Its the best tool for differentiating.

Let u = 1-x
Then apply the differential operator to both sides. So,

du = -1 dx
Now we want dx by itself. So we rearrange.

dx = -du

No you can substitute back to the integral.

Int(u^5 x-du) = Int(-u^5du)
= -u^6/6 + c
= -(1-x)^6/6 + c (answer)

IIRC, that's a 3U method

 

[turntaker]

I understand your method but this post is under mathematics. Reverse chain rule is more easier to understand and ultilise for a 2u student.

I would disagree. The differential operator is an easy concept really.
Maths should be seen as a skill not just a set of syllabus dot points you follow.

It doesn't hurt to know beyond the syllabus. In fact it can help you in the exam.
Personally I think the chain rule is not as powerful as this.

 

[Drsoccerball]

I would disagree. The differential operator is an easy concept really.
Maths should be seen as a skill not just a set of syllabus dot points you follow.

It doesn't hurt to know beyond the syllabus. In fact it can help you in the exam.
Personally I think the chain rule is not as powerful as this.

I still think the 2u method is easier as it is conventional...If it wasnt linear inside the brackets i would understand but for this definitely use reverse chain rule

 

[turntaker]

I still think the 2u method is easier as it is conventional...If it wasnt linear inside the brackets i would understand but for this definitely use reverse chain rule

yes true. However it is always best to have a method which works for everything.
Also I believe OP is also doing extension 1 so knowing this will definitely help.

 

[Fiction]

Thank you~

 

[photastic]

I would disagree. The differential operator is an easy concept really.
Maths should be seen as a skill not just a set of syllabus dot points you follow.

It doesn't hurt to know beyond the syllabus. In fact it can help you in the exam.
Personally I think the chain rule is not as powerful as this.

I think you should understand this post is in the 2u section, also in ext 1, they will give you a substitution. Also there are better methods than your one mentioned.

 

[turntaker]

I think you should understand this post is in the 2u section, also in ext 1, they will give you a substitution. Also there are better methods than your one mentioned.

Nah m9 my methods da bes

 

[enigma_1]

Why is there a debate on this lol
Turntaker go study for English

2u exam uses 2u method. Simple.

 

[turntaker]

I think you should understand this post is in the 2u section, also in ext 1, they will give you a substitution. Also there are better methods than your one mentioned.

Plz tell me the better methods

 

[turntaker]

Why is there a debate on this lol
Turntaker go study for English

2u exam uses 2u method. Simple.

We clearly aren't allowed to think outside the box here and introduce new ways of doing things.

 

[enigma_1]

We clearly aren't allowed to think outside the box here and introduce new ways of doing things.

Of course you are. Just do it in places where it's needed.

 

[turntaker]

My old grandfather always used to say "there is more than one way to skin a cat"
What a great chap he was.

 

[turntaker]

Of course you are. Just do it in places where it's needed.

Well I use this in 2u integration , 3u integration and 4u integration. It never fails.
Its s fool proof method.

Whereas the reverse product rule is good for specific cases and doesn't tend to work for harder problems.

 

[turntaker]

/thread closed guys soznotsoz