Geometrical Proofs using Vectors Questions (1 Viewer)

[csi]

Hi guys,

It'll be much appreciated if anyone could me with any of the below question. Thanks to all!!
Note: please prove all the below using vectors.

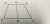
1. In any trapezium ABCD, show the length of MN (which connects the midpoints of the parallel sides of AB and CD) must be half the sum of AC and BD.

2. AB and CD are diameters of a circle with centre O. Prove that ABCD is a rectangle.

3. Prove that the lines joining the midpoints of opposite sides of a quadrilateral ABCD bisect each other.

4. Prove that the medians of a triangle meet at a point that divides each median in the ratio 2:1.

 

[CM_Tutor]

1. In any trapezium ABCD, show the length of MN (which connects the midpoints of the parallel sides of AB and CD) must be half the sum of AC and BD.

Is this meant to be that MN is parallel to AB and CD and connects the midpoints of the non-parallel sides?

Because otherwise, take AB as 3 units, CD as 5 units, then this theorem is saying that the length of MN must be 4... yet I can set the perpendicular height as any positive real, so if I make it 10 units, it's obvious that MN must be bigger than 4, and if I make the perpendicular height 100, it is clear that MN must be larger than in the former case, and so MN is not constant.

 

[CM_Tutor]

Some general advice:

1. Be careful in choosing which sides / intervals to name as vectors.
2. Name as few as you can so as to minimise the number of variables.
3. Think before defining - a good definition can make lifer easier.
4. Be clear on your goals.

To illustrate, consider question 2:

AB and CD are both diameters of the same circle, of centre O. Prove that ABCD is a rectangle.

First, notice that the question has mis-named the rectangle. It cannot be (in cyclic order) ABCD as AB and CD are diameters, meaning either C or D lies between A and B in one direction, and the other in the other direction. The rectangle must be ACBD (or ADBC, if you prefer).

Second, how do we prove a rectangle? Show one pair of opposite sides have the same vector and that any angle is a right angle.

So, we seek to show that and that

We could start by giving symbols to vectors for the sides, but that does not take account of the fact that we have a circle. So, I would define:

Let and . It immediately follows that and similarly that . And, since we have a circle, as these are equal radii.

You may notice that I did not use or or as vectors, to avoid confusion with vertices already including and and while is commonly used for a radius, in this case it could be confusing as to which is meant.

Now,

And,

So, we have one pair of opposite sides having the same vector, and so being equal in length and parallel, so we have proven our quadrilaterial is a parallelogram, and the first part of the proof is complete.

Further,

Thus,



We have shown that the angle between vectors and is a right angle, and so one angle of the parallelogram is a right angle, and thus the parallelogram is a rectangle.

 

[csi]

Some general advice:

1. Be careful in choosing which sides / intervals to name as vectors.
2. Name as few as you can so as to minimise the number of variables.
3. Think before defining - a good definition can make lifer easier.
4. Be clear on your goals.

To illustrate, consider question 2:

AB and CD are both diameters of the same circle, of centre O. Prove that ABCD is a rectangle.

First, notice that the question has mis-named the rectangle. It cannot be (in cyclic order) ABCD as AB and CD are diameters, meaning either C or D lies between A and B in one direction, and the other in the other direction. The rectangle must be ACBD (or ADBC, if you prefer).

Second, how do we prove a rectangle? Show one pair of opposite sides have the same vector and that any angle is a right angle.

So, we seek to show that and that

We could start by giving symbols to vectors for the sides, but that does not take account of the fact that we have a circle. So, I would define:

Let and . It immediately follows that and similarly that . And, since we have a circle, as these are equal radii.

You may notice that I did not use or or as vectors, to avoid confusion with vertices already including and and while is commonly used for a radius, in this case it could be confusing as to which is meant.

Now,

And,

So, we have one pair of opposite sides having the same vector, and so being equal in length and parallel, so we have proven our quadrilaterial is a parallelogram, and the first part of the proof is complete.

Further,

Thus,



We have shown that the angle between vectors and is a right angle, and so one angle of the parallelogram is a right angle, and thus the parallelogram is a rectangle.

Thanks

 

[csi]

Is this meant to be that MN is parallel to AB and CD and connects the midpoints of the non-parallel sides?

Because otherwise, take AB as 3 units, CD as 5 units, then this theorem is saying that the length of MN must be 4... yet I can set the perpendicular height as any positive real, so if I make it 10 units, it's obvious that MN must be bigger than 4, and if I make the perpendicular height 100, it is clear that MN must be larger than in the former case, and so MN is not constant.

I’m not sure, but this is the diagram they provided. The letters on there are just me trying to figure out the question.

 

[CM_Tutor]

I’m not sure, but this is the diagram they provided. The letters on there are just me trying to figure out the question.

Ok, thanks.

There is a simple answer. The question is wrong.

Disproof of the question given:

Consider their diagram and take the point that they have labelled as as the origin, so that the direction from to corresponds to the direction. I can add a set of axes like this without loss of generality.

Now, since the result (if true), applies to any trapezium, I can choose one of a convenient scale and know the argument holds for any similar trapezium. So, I choose to define:

• Let
• Since with and having the same direction, but with , so let
• Let's take and let , an acute angle (in line with the diagram)

Simple vector calculations should confirm that

With as the midpoint of and as the midpoint of , it follows that:



Recall, we seek to prove that



where



and we have just shown that



These cannot possibly have the same value for all acute angles and so the theorem cannot be true generally. In fact, for the trapezium that has been chosen, for the result to be true, we would need



and since this equation has no real solution, the required property is never true for a trapezium with longer parallel side being three times the shorter and with the shorter located over the longer so that is an acute angle.

---

Looking at the diagram again, however, and taking account of the scale, I could define the origin at so that:

• 
• 
• 

and see if the result works for this specific trapezium. But... it doesn't.

---

However, the version that I suggested is true (and in general).

Let be a trapezium in which . Let and be the midpoints of and , respectively. Show that



by showing that