any suggestions about how to approach this question on continuous pdf? (1 Viewer)
- Thread starter Fizsi
- Start date
I think that @Fizsi means the piece of the function for 
to be
 = \frac{1}{x+a})
rather than
 = \frac{1}{x}+a)
because, as @cossine has noted, this second interpretation leads to no solution being possible.
Assuming the first interpretation, for
, we have
}=\lim_{x \to 0^-}{(x+a)}=0+a=a)
and for, we have
}=\lim_{x \to 0^+}{\frac{1}{x+a}} = \frac{1}{0+a} = \frac{1}{a})
For)
to be continuous, the branches must meet and the limits must be the same, and thus:
.
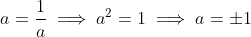
rather than
because, as @cossine has noted, this second interpretation leads to no solution being possible.
Assuming the first interpretation, for
and for
For