Arg qs. (1 Viewer)

ExtremelyBoredUser · Nov 6, 2021

The normal way for Q.22 is to simply do a vector diagram and it might even be better;

$|w_2| = |w_1|$ however $arg(w_2) > arg(w_1)$ so when you draw these vectors, you would get w_1 vector having a larger argument than w_2 but the same distance. You should realise that you can create a parallelogram.

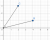
Sample of a graph you should draw (not to scale)

Through parallelogram law.

AB + AC = AD
and AD bisects both AB and AC so therefore
$arg(w_2+w_1) = \frac{1}{2} (arg(w_1) + arg(w_2))$

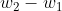$w_2 - w_1$ is simply CD and you can do this through vector addition.

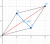
As you can see, the vector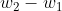 $w_2 - w_1$ is perpendicular to the vector $w_2 + w_1$ and hence there is a right angle subtended.

Therefore

$arg(w_2 - w_1) = arg(w_2 + w_1) - \frac{\pi}{2}$
$arg(w_2 - w_1) = \frac{\alpha_1 + \alpha_ 2 - \pi}{2}$

As required. This is the proper way I presume you are supposed to answer the question as this is under the vectors chapter and it is simply just geometric reasoning however I also used another way which might not be as proper/conventional but nonetheless is another way.

Method 2:

$w_1 = |w_1|e^{i\alpha_1} = e^{i\alpha_1$ as both modulus are equal to 1
$w_2 = |w_2|e^{i\alpha_2} = e^{i\alpha_2}$

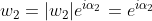
By definition of complex numbers. You can write them in mod-arg form if that is more clearer.

$w_1 - w_2 = e^{i\alpha_2} - e^{i\alpha_1$
$e^{\frac{\alpha_1 + \alpha_2}{2}} * ( e^{i\frac{\alpha_1 - \alpha_2}{2} - e^i{\frac{\alpha_2 - \alpha_1}{2}} )$
$e^{\frac{\alpha_1 + \alpha_2}{2}} * -2isin(\theta)$ Let theta just be the argument of $\frac{\alpha_2 - \alpha_1}{2}$
$e^{\frac{\alpha_1 + \alpha_2}{2}} * e^{i\frac{-\pi}{2}} 2sin(\theta)$ converting - i into eulers form (you can do this in mod arg remember)
$e^{\frac{\alpha_1 + \alpha_2 -\pi}{2}} * 2sin(\theta)$

As you can see now, it is in the complex number form as I showed above.
hence
$arg(w_1 - w_2) = \frac{\alpha_1 + \alpha_2 -\pi}{2}$

YourLocalDumbAss · Nov 6, 2021

ExtremelyBoredUser said:
The normal way for Q.22 is to simply do a vector diagram and it might even be better;

$|w_2| = |w_1|$ however $arg(w_2) > arg(w_1)$ so when you draw these vectors, you would get w_1 vector having a larger argument than w_2 but the same distance. You should realise that you can create a parallelogram.
View attachment 33418
Sample of a graph you should draw (not to scale)

View attachment 33419
Through parallelogram law.

AB + AC = AD
and AD bisects both AB and AC so therefore
$arg(w_2+w_1) = \frac{1}{2} (arg(w_1) + arg(w_2))$

$w_2 - w_1$ is simply CD and you can do this through vector addition.

View attachment 33420

As you can see, the vector $w_2 - w_1$ is perpendicular to the vector $w_2 + w_1$ and hence there is a right angle subtended.

Therefore

$arg(w_2 - w_1) = arg(w_2 + w_1) - \frac{\pi}{2}$
$arg(w_2 - w_1) = \frac{\alpha_1 + \alpha_ 2 - \pi}{2}$

As required. This is the proper way I presume you are supposed to answer the question as this is under the vectors chapter and it is simply just geometric reasoning however I also used another way which might not be as proper/conventional but nonetheless is another way.

Method 2:

$w_1 = |w_1|e^{i\alpha_1} = e^{i\alpha_1$ as both modulus are equal to 1
$w_2 = |w_2|e^{i\alpha_2} = e^{i\alpha_2}$

By definition of complex numbers. You can write them in mod-arg form if that is more clearer.

$w_1 - w_2 = e^{i\alpha_2} - e^{i\alpha_1$
$e^{\frac{\alpha_1 + \alpha_2}{2}} * ( e^{i\frac{\alpha_1 - \alpha_2}{2} - e^i{i\frac{\alpha_2 - \alpha_1}{2}} )$
$e^{\frac{\alpha_1 + \alpha_2}{2}} * -2isin(\theta)$ Let theta just be the argument of $\frac{\alpha_2 - \alpha_1}{2}$
$e^{\frac{\alpha_1 + \alpha_2}{2}} * e^{i\frac{-\pi}{2}} 2sin(\theta)$ converting - i into eulers form (you can do this in mod arg remember)
$e^{\frac{\alpha_1 + \alpha_2 -\pi}{2}} * 2sin(\theta)$

As you can see now, it is in the complex number form as I showed above.
hence
$arg(w_1 - w_2) = \frac{\alpha_1 + \alpha_2 -\pi}{2}$

eddie woo, take that mask off NOW!

5uckerberg · Nov 6, 2021

For Q22 the work is cut out for you.

=)(= said:
View attachment 33414

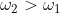For Q22 this will be my explanation. From the diagram given one can say that $\omega_{2}>\omega_{1}$ and that using vector addition you will see what I was going to bring up. Ah yes, I also was going to bring up the average of the two arguments as well but Extremely Bored User bought it up using the parallelogram law so thanks for that. Anyways this was my approach to this question.

=)(= · Nov 7, 2021

thank you very much

for this how did they get rid of the -1 from squaring i

5uckerberg · Nov 7, 2021

Conjugate root theorem which is $|z|^{2}=z\bar{z}$ where $\bar{z}$ has a negative version of the imaginary part of the complex number.

For future students of Mathematics, Extension II please note that suppose we have an imaginary number calling it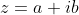 $z=a+ib$ and that $|z|^{2}=a^{2}+b^{2}$ what is happening is that we are using the fact that $|z|^{2}=z\bar{z}=|(a+ib)|^{2}=(a+ib)(a-ib)=a^{2}+b^{2}-aib+aib=a^{2}+b^{2}$ .

Please play around with this idea until it becomes second nature

=)(= · Nov 7, 2021

ohh makes sense

=)(= · Nov 7, 2021

How would you do this. I get argz-argz+4 =3pi/4 meaning the angle between the two points is 3pi/4 but i don't understand how a section of a circle is produced from that

ExtremelyBoredUser · Nov 7, 2021

=)(= said:
View attachment 33429
How would you do this. I get argz-argz+4 =3pi/4 meaning the angle between the two points is 3pi/4 but i don't understand how a section of a circle is produced from that

$arg(z) = \frac{3\pi}{4} + arg(z+4)$

General rule is that you start from the LHS, so arg(z) and you move anticlockwise till you reach the other point in RHS so arg(z+4).

This means you would start at point 0 from arg(z) and move anticlockwise to point -4 as from arg(z+4).

This is the desired locus but you can't really tell it from first glance.

So to recognise its a section;

note that the angle at the centre is double of the angle at the circumference. I forgot what the name is but that is one property. 3pi/4 * 2 = 3pi/2 but that is over the domain for a triangle so subtracting by 2 you should get -pi/2 which means the angle will be under the axis.

$\angle AFB = \frac{3\pi}{4}$ where F is some arbitrary point on the locus as given by the arg equation
$\angle EAB= \frac{\pi}{2}$ where C is the centre of the circle.

Now angle AFB doesn't really help much but angle EAB will. Recognise that the centre must have an angle of 90 degrees when connecting from A and B.

You would first draw the line from A or B to a centre point (which you wouldnt know)

You wouldn't know exactly the points as you wouldn't use a graphing software but the diagram should be something like this. 90 degrees on angle AEB. Now you can deduce that the angles for triangle ADE is 90,45,45 through the diagram and vice versa with EBD.

You already know the midpoint is -2 so therefore the vector AD would have a magnitude of 2. Using trig you can find the vector AE which will consequently be the radius.

$cos(45) = 2/r$
$r = 2/cos(45)$
$r = \sqrt(8)$

Likewise you can find the point of the centre through trig.

The centre would simply be the distance of vector DE.

Pythagoras theorem would give you 2 and you can just find the coordinate of E through going 2 down from D.

So E = (-2,-2)

Now you can draw out the region through.

$(x-2)^2 + (y-2)^2 = 8$

is the equation of the circle.

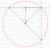
Of course only the region from point 0 to point -4 anticlockwise should be coloured in and the rest should be lined.

You wouldn't need to go to this much depth/effort but its good to understand whats going on before you do the region. For me, I can recognise when it will be a section of the circle as required as given by the equation of the arg but thats the reasoning I used to convince myself, there might be a better way to address this but its what made sense for me.

Life'sHard · Nov 7, 2021

=)(= said:
View attachment 33429
How would you do this. I get argz-argz+4 =3pi/4 meaning the angle between the two points is 3pi/4 but i don't understand how a section of a circle is produced from that

5uckerberg · Nov 10, 2021

=)(= said:
View attachment 33429
How would you do this. I get argz-argz+4 =3pi/4 meaning the angle between the two points is 3pi/4 but i don't understand how a section of a circle is produced from that

The first thing one would need to do is to note that $arg(\frac{z}{z+4})=arg(z)-arg(z+4)$ and also to start off consider for this Q $arg(z)=\frac{3\pi}{4}$ and $arg(z+4)=0$ . That should really be your starting point because you do not need to deal with the second term because we as mathematicians do not want to give ourselves too many complications. Next, using the fact that external angle is the sum of the two internal angles where the external angle is $\frac{3\pi}{4}$ and by default, the two internal angles have to equal that.

A common way to tackle this type of question is instead of using graphing software as ExtremelyBoredUser said you can observe a sunset where the sun is gradually descending below the horizon of our eyes and we can use that to find the locus around the edge of the sun. Go and watch some sunsets when the weather is nice and visually draw the locus around the sun with your finger. This is a helpful way to gain an intuition for questions that require us to sketch the locus of the argument.

CM_Tutor · Nov 11, 2021

=)(= said:
View attachment 33429
How would you do this. I get argz-argz+4 =3pi/4 meaning the angle between the two points is 3pi/4 but i don't understand how a section of a circle is produced from that

$\arg\left(\cfrac{z}{z+4}\right)=\cfrac{3\pi}{4}$

While you are looking at the locus of points $P$ being described, you need to remember that a point on its own has no argument. To have an argument, and thus direction, you need at least two points or a line or vector. Rewriting this as

$\arg{z}-\arg{(z+4)}=\cfrac{3\pi}{4}$

involves $\arg{z}$ , which refers to the direction in which the point $z$ is located from the origin. $\arg{(z+4)} = \arg{(z - -4)}$ similarly refers to the direction in which the point $z$ is located from the point -4 on the real axis.

So, draw co-ordinates axes, label the origin as $O$ and the point -4 as $A$ . Put a point $P$ somewhere in the first quadrant. Label some point on the positive real axis as $X$ . Now, joining $O$ to $P$ , can you see that $\angle{POX} = \alpha = \arg{z}$ ? And, joining $A$ to $P$ , can you see that $\angle{PAX} = \beta = \arg{(z+4)}$ ? Does it follow that $\angle{APO} = \alpha - \beta$ ? Trying different places for $P$ , you should find that $\angle{APO}$ is either $\alpha - \beta$ or $\beta - \alpha$ so long as $P$ is not at $O$ or $A$ .

Your locus is then the set of points $P$ such that the angle between the vectors is (in this case) $\cfrac{3\pi}{4}$ .

Now, if the locus had been $\arg\left(\cfrac{z}{z+4}\right)=\cfrac{\pi}{2}$ then $P$ would lie on the circle of which $OA$ is diameter. Attempting to solve the problem algebraically would yield $(x-2)^2 + y^2 = 4$ . However, the points $O$ and $A$ are excluded (as one of the arguments is undefined). Furthermore, the portion of the circle below the real axis has $\arg\left(\cfrac{z}{z+4}\right)=-\cfrac{\pi}{2}$ , and the locus is only the part above the real axis with open circles at $O$ and $A$ . It is easier to see which part of the circle is included and which part is excluded from the diagram than by doing the algebra.

For $\arg\left(\cfrac{z}{z+4}\right)=\phi$ for any angle $0 \leqslant |\phi| \leqslant \pi,\ \phi \neq \pm\cfrac{\pi}{2}$ , the locus will be part of a circle of which $AO$ is a chord, with a centre somewhere on $\Re{(z)}=-2,\ \Im{(z)}\neq 0$ .

If $\phi = 0$ , the locus will be the real axis excluding the interval $OA$ (and excluding the points $O$ and $A$ ), because $\arg{z} = \arg{(z+4)}$ is saying that the points $P$ are located where the direction from $O$ and from $A$ is the same.

If $\phi = \pm\pi$ . the locus will be the interval $OA$ (though still excluding the points $O$ and $A$ ) because $\arg{z} - \arg{(z+4)} = \pi$ is saying that the points $P$ are located in the exact opposite directions from $O$ and from $A$ , and thus must be collinear with, and between, points $O$ and $A$ .

YourLocalDumbAss · Nov 11, 2021

Life'sHard said:

@ExtremelyBoredUser’s identity has been leaked

=)(= · Nov 12, 2021

how would you go about doing ci and cii?

5uckerberg · Nov 12, 2021

=)(= said:
how would you go about doing ci and cii?
View attachment 33594

Part a is quite easy to dissect. Use De Moivre's theorem, which should give us $\cos^{7}\theta-21\cos^{5}\theta+35\cos^{3}\theta-7\cos{\theta}$
Part b is quite easy as well all you have to do is let $\cos{7\theta}=0, \theta=\frac{\pi}{14}, \frac{3\pi}{14}, \frac{5\pi}{14}$ as such $x=4\cos^{2}\frac{\pi}{14}, 4\cos^{2}\frac{3\pi}{14}, 4\cos^{2}\frac{5\pi}{14}$ .
Part c what is actually happening there is suppose we turn $4\cos^{2}\frac{\pi}{14}=\alpha, 4\cos^{2}\frac{3\pi}{14}=\beta, 4\cos^{2}\frac{5\pi}{14}=\gamma$ . Then the sum of roots is $\alpha+\beta+\gamma=\frac{-b}{a}=\frac{-(-7)}{1}=7$ . But we want $\cos^{2}\frac{\pi}{14}+\cos^{2}\frac{3\pi}{14}+\cos^{2}\frac{5\pi}{14}$ . There the simplest way to get there is to divide the sum of roots for x by 4 giving us $\cos^{2}\frac{\pi}{14}+\cos^{2}\frac{3\pi}{14}+\cos^{2}\frac{5\pi}{14}=\frac{7}{4}$ .

part d is another elegent one. This part if you have learnt inequalities well you will be able to observe that $(\alpha+\beta+\gamma)^{2}=\alpha^{2}+\beta^{2}+\gamma^{2}+2\alpha\beta+2\beta\gamma+2\gamma\alpha$ . If you want that to be raised to the power of 4 replace $(\cos{x}+\cos{y}+\cos{z})^{2}=\cos^{2}{x}+\cos^{2}{y}+\cos^{2}{z}+2\cos{x}\cos{y}+2\cos{y}\cos{z}+2\cos{z}\cos{x}$ with $(\cos^{2}x+\cos^{2}y+\cos^{2}z)^{2}=\cos^{4}x+\cos^{4}y+\cos^{4}z+2\cos^{2}x\cos^{2}y+2\cos^{2}y\cos^{2}z+2\cos^{2}z\cos^{2}x$ . Now note that $2\cos^{2}x\cos^{2}y+2\cos^{2}y\cos^{2}z+2\cos^{2}z\cos^{2}x$ is in reality $2(\cos^{2}{x}\cos^{2}{y}+\cos^{2}{y}\cos^{2}{z}+\cos^{2}{z}\cos^{2}{x})$ which can then be thought of as $2(\frac{\alpha\beta}{16}+\frac{\gamma\beta}{16}+\frac{\alpha\gamma}{16})=\frac{\alpha\beta+\gamma\beta+\gamma\beta}{8}=\frac{14}{8}$ using the fact that sum of the product of two roots is $\frac{c}{a}$ in a given polynomial $ax^{3}+bx^{2}+cx+d$ . Next we want to find $\cos^{4}x+\cos^{4}y+\cos^{4}z=\frac{49}{16}-\frac{28}{16}=\frac{21}{16}.$

Arg qs. (1 Viewer)

=)(=

Active Member

ExtremelyBoredUser

Bored Uni Student

YourLocalDumbAss

Well-Known Member

5uckerberg

Well-Known Member

Attachments

=)(=

Active Member

5uckerberg

Well-Known Member

=)(=

Active Member

=)(=

Active Member

ExtremelyBoredUser

Bored Uni Student

Life'sHard

Well-Known Member

5uckerberg

Well-Known Member

CM_Tutor

Moderator

YourLocalDumbAss

Well-Known Member

=)(=

Active Member

5uckerberg

Well-Known Member

Users Who Are Viewing This Thread (Users: 0, Guests: 1)