How do you determine the graph shape for complex number locuses? (1 Viewer)

[sadpwner]

It's always a multiple question that gives you

modulus(z+x+iy) plus or minus mod(z+b+in)

In what cases is it a:
circle
eclipse
hyperbola
straight line

 

[braintic]

You are going to have to write this more clearly.
Is there one modulus or two?
And if it is a locus, it must be an equation. Where is the equal sign?

 

[glittergal96]

The ones involving just distances to two points that are good to recognise:



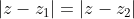
(the line that bisects z1z2).



(the circle with diameter given by the line segment joining the internal and external points of k:1 division).



(the ellipse with focii at z1 and z2 with semimajor axis length k/2).



(the hyperbola with focii at z1 and z2 with distance between branches k/2).


You should also recognise the ones comparing distance to a point and distance to a line, like



(the conic with eccentricity e that has a focus at z1 and the x-axis as its corresponding directrix.)

 

[braintic]

The ones involving just distances to two points that are good to recognise:



(the line that bisects z1z2).



(the circle with diameter given by the line segment joining the internal and external points of k:1 division).



(the ellipse with focii at z1 and z2 with semimajor axis length k/2).



(the hyperbola with focii at z1 and z2 with distance between branches k/2).


You should also recognise the ones comparing distance to a point and distance to a line, like



(the conic with eccentricity e that has a focus at z1 and the x-axis as its corresponding directrix.)

For your 3rd example, I don't think k>0 is sufficiently restrictive in order to get an ellipse.

 

[glittergal96]

For your 3rd example, I don't think k>0 is sufficiently restrictive in order to get an ellipse.

Ah yes, neither for the hyperbola.

We require k > |z1-z2| for the ellipse and k < |z1-z2| for the hyperbola to avoid degeneracy.

 

[braintic]

Ah yes, neither for the hyperbola.

We require k > |z1-z2| for the ellipse and k < |z1-z2| for the hyperbola to avoid degeneracy.

And ... if you'll allow me to be even more picky (for the sake of OP, not you):

In the 2nd we need k not equal to 1 (if we really want a circle).

And for the last one, you probably want an absolute value around the Im(z), unless you specify Im(z_1) > 0 for an ellipse, or unless you want only one branch of a hyperbola.

 

[glittergal96]

And ... if you'll allow me to be even more picky (for the sake of OP, not you):

In the 2nd we need k not equal to 1 (if we really want a circle).

And for the last one, you probably want an absolute value around the Im(z), unless you specify Im(z_1) > 0 for an ellipse, or unless you want only one branch of a hyperbola.

Yep, by all means.

Cheers, will amend a little later.