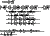-
Looking for HSC notes and resources? Check out our Notes & Resources page
Make a Difference – Donate to Bored of Studies!
Students helping students, join us in improving Bored of Studies by donating and supporting future students!
HSC 2012 MX1 Marathon #2 (archive) (1 Viewer)
- Thread starter bleakarcher
- Start date
Timske
Sequential
- Joined
- Nov 23, 2011
- Messages
- 794
- Gender
- Male
- HSC
- 2012
- Uni Grad
- 2016
Re: HSC 2012 Marathon 
is it meant to be sin54cos26?Not sure if this question works but oh well
=\sin54 \cos26-\sin26 \sin36. $ Give the answer in degrees. $)
Sy123
This too shall pass
- Joined
- Nov 6, 2011
- Messages
- 3,725
- Gender
- Male
- HSC
- 2013
Re: HSC 2012 Marathon 
=\sin54 \cos26 -sin26 \cos54 \\ \\ \cos(3\theta +2)=\sin(28) \\ \\ 3\theta +2=360k \pm cos^{-1}(sin28) \\ \\ \therefore \theta=\frac{1}{3} (360k \pm cos^{-1}(sin28)-2) )
Ok time for my question:
^n \\ \\ $Prove that$ \\ \\ e=1+\frac{1}{1!}+\frac{1}{2!}+\frac{1}{3!}+... )
Have fun!
Not sure if this question works but oh well
=\sin54 \cos26-\sin26 \sin36. $ Give the answer in degrees. $)
Ok time for my question:
Have fun!
Last edited:
asianese
Σ
- Joined
- Sep 20, 2010
- Messages
- 2,219
- Gender
- Undisclosed
- HSC
- 2012
Re: HSC 2012 Marathon 
Having a stab at that one...It's probably fudged...but just trying.
^n\\ \begin{align*} \left( 1+\frac{1}{n} \right)^n &= \binom{n}{0}+\binom{n}{1} \left(\frac{1}{n}\right)+\binom{n}{2} \left(\frac{1}{n}\right)^2+\binom{n}{3} \left(\frac{1}{n}\right)^3+\ldots + \binom{n}{n} \left(\frac{1}{n}\right)^n\\ &1+\frac{n!}{1!(n-1)!n}+\frac{n!}{2!(n-2)!n^2}+\frac{n!}{3!(n-3)!n^3}+\ldots \ldots \\ &=1+\frac{1}{1!}+\frac{n-1}{2!n}+\frac{(n-1)(n-2)}{3!n^2}+\ldots \ldots \\ &=1+\frac{1}{1!}+\frac{1}{2!}\left(1+\frac{1}{n}\right)+\frac{1}{3!}\left(\frac{n^2-3n+2}{n^2}\right)+\ldots \ldots \\ &=1+\frac{1}{1!} + \frac{1}{2!}\left(1+\frac{1}{n}\right)+\frac{1}{3!}\left(1-\frac{3}{n}+\frac{2}{n^2}\right)+\ldots \ldots \\ \end{align*} \\ $Consider $ \lim_{n \to \infty}\left(1+\frac{1}{n}\right)^n= 1+\frac{1}{1!}+\frac{1}{2!}(1+0)+\frac{1}{3!}(1-0-0)+\ldots \ldots \\ = 1+\frac{1}{1!}+\frac{1}{2!}+\frac{1}{3!}+\ldots \ldots)
Ok the equation works on codecogs perfectly but doesn't here. WTF
Having a stab at that one...It's probably fudged...but just trying.
Ok the equation works on codecogs perfectly but doesn't here. WTF
Attachments
-
11.6 KB Views: 61
Last edited:
Carrotsticks
Retired
- Joined
- Jun 29, 2009
- Messages
- 9,467
- Gender
- Undisclosed
- HSC
- N/A
Re: HSC 2012 Marathon 
Too hard checking the first chunk of TeX =(
Tried to fix for you.Having a stab at that one...It's probably fudged...but just trying.
^n= 1+\frac{1}{1!}+\frac{1}{2!}(1+0)+\frac{1}{3!}(1-0-0)+\ldots \ldots \\ = 1+\frac{1}{1!}+\frac{1}{2!}+\frac{1}{3!}+\ldots \ldots\\ $Hence $\begin{align*} e&=\lim_{n\to \infty}\left(1+\frac{1}{n} \right)^n &= 1+\frac{1}{1!}+\frac{1}{2!}+\ldots \ldots\end{align*})
Too hard checking the first chunk of TeX =(
Last edited:
asianese
Σ
- Joined
- Sep 20, 2010
- Messages
- 2,219
- Gender
- Undisclosed
- HSC
- 2012
Re: HSC 2012 Marathon 
Don't worry carrot lol. For some reason lately my upload speed has been terrible so I can't even upload a simple gif i downloaded off codecogs.
ANYWAY
What I did was Consider (1+1/n)^n and expand with binomial theorem: Cn0 +Cn1(1/n)+Cn2(1/n^2)=...
Then expand out into factorial notation
= 1+ n!/1!(n-1)! * 1/n ...
=1+ 1/1! + (n-1)/2!*n +...
=1+1/1! + (1/2!)* (1+1/n) and the rest of the brackets end up with 1 +/- x/n, n^2 so they all disappear once we take the limit as n=> infinity since 1/n goes off to 0. So you're left with 1+1/1!+1/2!+1/3!... which is the required result.
Don't worry carrot lol. For some reason lately my upload speed has been terrible so I can't even upload a simple gif i downloaded off codecogs.
ANYWAY
What I did was Consider (1+1/n)^n and expand with binomial theorem: Cn0 +Cn1(1/n)+Cn2(1/n^2)=...
Then expand out into factorial notation
= 1+ n!/1!(n-1)! * 1/n ...
=1+ 1/1! + (n-1)/2!*n +...
=1+1/1! + (1/2!)* (1+1/n) and the rest of the brackets end up with 1 +/- x/n, n^2 so they all disappear once we take the limit as n=> infinity since 1/n goes off to 0. So you're left with 1+1/1!+1/2!+1/3!... which is the required result.
seanieg89
Well-Known Member
- Joined
- Aug 8, 2006
- Messages
- 2,653
- Gender
- Male
- HSC
- 2007
Re: HSC 2012 Marathon 
lim (1+1/n)^n = lim 1^n = 1, as each term in the n-fold product (1+1/n)(1+1/n)...(1+1/n) tends to 1.
A classic fallacious argument in analysis. The number of terms in this series is not fixed, so we cannot simply take the limits of each term individually and add them. If things like this were legit then we would have for exampleDon't worry carrot lol. For some reason lately my upload speed has been terrible so I can't even upload a simple gif i downloaded off codecogs.
ANYWAY
What I did was Consider (1+1/n)^n and expand with binomial theorem: Cn0 +Cn1(1/n)+Cn2(1/n^2)=...
Then expand out into factorial notation
= 1+ n!/1!(n-1)! * 1/n ...
=1+ 1/1! + (n-1)/2!*n +...
=1+1/1! + (1/2!)* (1+1/n) and the rest of the brackets end up with 1 +/- x/n, n^2 so they all disappear once we take the limit as n=> infinity since 1/n goes off to 0. So you're left with 1+1/1!+1/2!+1/3!... which is the required result.
lim (1+1/n)^n = lim 1^n = 1, as each term in the n-fold product (1+1/n)(1+1/n)...(1+1/n) tends to 1.
Carrotsticks
Retired
- Joined
- Jun 29, 2009
- Messages
- 9,467
- Gender
- Undisclosed
- HSC
- N/A
Re: HSC 2012 Marathon 
A little iffy about that question, because the NSW Syllabus only ever defines integral values of n (for the Binomial Expansion)...
Have fun!
seanieg89
Well-Known Member
- Joined
- Aug 8, 2006
- Messages
- 2,653
- Gender
- Male
- HSC
- 2007
Re: HSC 2012 Marathon 
 , but it is sort of uni analysis-y.
, but it is sort of uni analysis-y.
That is all this question needsA little iffy about that question, because the NSW Syllabus only ever defines integral values of n (for the Binomial Expansion)...
Sy123
This too shall pass
- Joined
- Nov 6, 2011
- Messages
- 3,725
- Gender
- Male
- HSC
- 2013
Re: HSC 2012 Marathon 
Im sorry if its not that good of a question, I just thought I would be a little creative rather than grabbing some question from a trial or textbook or something. :/
But either way, great work asianese, that is, if that proof is valid, I did something slightly differently.
I proved the limit of the binomial expansion's first few terms is 1+1/1!+1/2!
Then I proved it for integer 'r'
!r!} \times \frac{1}{n^r} \\ \\ \frac{n(n-1)(n-2)...(n-r+1)}{r! n^r} \\ \\ \frac{(n-1)(n-2)...(n-r+1)}{r!n^{r-1}} \\ \\ \frac{n^{r-1}}{r! n^{r-1}}+\frac{k n^{r-2}}{r! n^{r-1}}+\frac{k_1 n^{r-3}}{r! n^{r-1}}+...+\frac{k_2}{r! n^{r-1}} \\ \\ as \ \ n \to \infty \\ \\ \frac{1}{r!}+0+0+... \\ \\ \therefore $The binomial expansion of the limit, will work out for the rth term of any integer r$ )
So I proved it for any integer r as well, however if any of the math pros could tell us the problem with our solution, and if so, is there a 3u solution possible for this proof, because this is the proof I had in mind when I asked it.
Thanks!
Im sorry if its not that good of a question, I just thought I would be a little creative rather than grabbing some question from a trial or textbook or something. :/
But either way, great work asianese, that is, if that proof is valid, I did something slightly differently.
I proved the limit of the binomial expansion's first few terms is 1+1/1!+1/2!
Then I proved it for integer 'r'
So I proved it for any integer r as well, however if any of the math pros could tell us the problem with our solution, and if so, is there a 3u solution possible for this proof, because this is the proof I had in mind when I asked it.
Thanks!
asianese
Σ
- Joined
- Sep 20, 2010
- Messages
- 2,219
- Gender
- Undisclosed
- HSC
- 2012
Re: HSC 2012 Marathon 
Yeah I also had a little suspicion because I didn't know what term to do it up to. If I did it to the nth term that would mean that the nth term disappears and there isn't any n! left off... Oh well, learn something everyday.
Yeah I also had a little suspicion because I didn't know what term to do it up to. If I did it to the nth term that would mean that the nth term disappears and there isn't any n! left off... Oh well, learn something everyday.
seanieg89
Well-Known Member
- Joined
- Aug 8, 2006
- Messages
- 2,653
- Gender
- Male
- HSC
- 2007
Re: HSC 2012 Marathon 
This is the problem with your argument Sy123, you cannot treat such a series "term by term". There isn't really a proof of this equality that a 3U student could be expected to produce...I will post a sandwich argument a bit later.A classic fallacious argument in analysis. The number of terms in this series is not fixed, so we cannot simply take the limits of each term individually and add them. If things like this were legit then we would have for example
lim (1+1/n)^n = lim 1^n = 1, as each term in the n-fold product (1+1/n)(1+1/n)...(1+1/n) tends to 1.