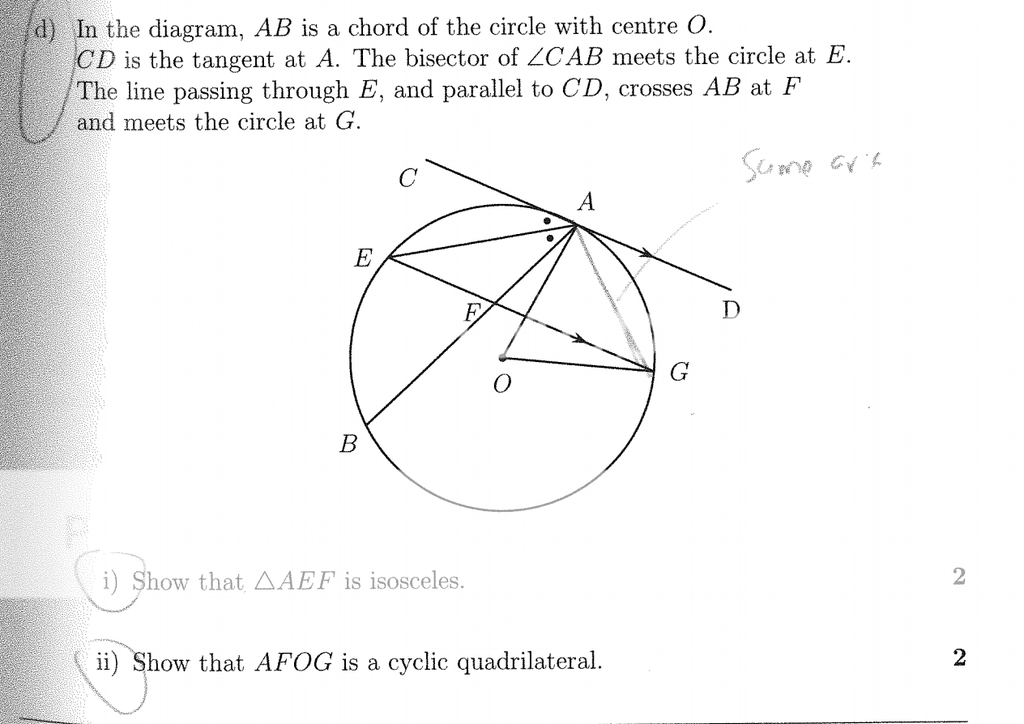
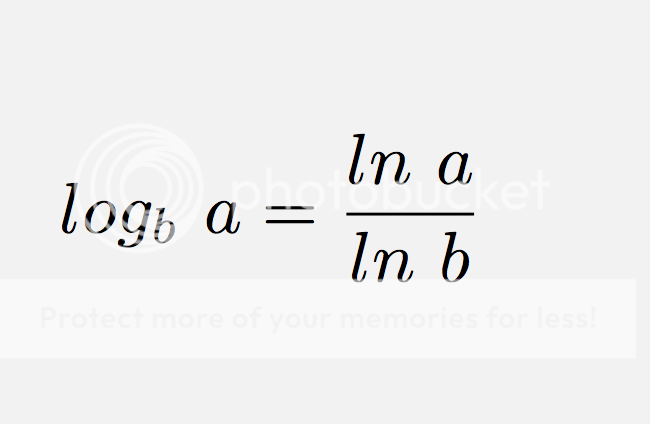davidgoes4wce
Well-Known Member
Re: HSC 2015 3U Marathon
Have learnt this course for nearly 12 months now ( did the prelim for 6 months). My slight weakness is Binomial Theorem and Geometry.
Its not that I haven't practiced enough but I kind of went through 4/5 of the book in Fitzpatrick and did zilch form Margaret Grove in Prelim and HSC texts.
I will make sure I go over Grove and the concepts again in summer.
Have learnt this course for nearly 12 months now ( did the prelim for 6 months). My slight weakness is Binomial Theorem and Geometry.
Its not that I haven't practiced enough but I kind of went through 4/5 of the book in Fitzpatrick and did zilch form Margaret Grove in Prelim and HSC texts.
I will make sure I go over Grove and the concepts again in summer.