Integration volume question (1 Viewer)

[nazfiz]

Find the volume of the solid formed when the region bounded by the parabola, y=9-x^2 and the co-ordinate axes is rotated a) about the X-axis b) about the Y-axis

Thank in advance, working out would be appreciated.

 

[bleakarcher]

y=9-x^2
a) V=pi*integral[(9-x^2)^2] dx from x=0 to x=3
V=pi*integral[81-18x^2+x^4] dx from x=0 to x=3
V=pi[81x-6x^3+(1/5)x^5] from x=0 to x=3
V=648pi/5 u^3
b) x^2=9-y
V=pi*integral[9-y] dy from y=0 to y=9
V=pi[9y-(1/2)y^2] from y=0 to y=9
V=pi[9*9-(1/2)*9^2]=81pi/2 u^3

sorry for the confusion, i have edited

 

[nazfiz]

Thanks man

 

[bleakarcher]

no worries dude, btw ur in year 10, y r u asking this? (u can call me a hyprocrite i guess for saying as i am in year 10 too answering it lol)

 

[nightweaver066]

I see you're working pretty far ahead.

First, draw a graph so you know what you're working with.

a) Volume of shaded region rotated about x axis is given by:










b)
Volume of shaded area rotated around the y-axis is given by:

 

[bleakarcher]

disregard my answer to the first one, i ended up integrating [9-x^2] not [9-x^2]^2 lol, i need to learn latex much clearer...

 

[bleakarcher]

nightweaver, u didnt realise the area to be rotated was bounded by the curve and the ordinate axis ie the x and y axis

 

[nightweaver066]

nightweaver, u didnt realise the area to be rotated was bounded by the curve and the ordinate axis ie the x and y axis

Misread the question lol. Will edit my post a bit.

 

[nazfiz]

Thanks guys

no worries dude, btw ur in year 10, y r u asking this? (u can call me a hyprocrite i guess for saying as i am in year 10 too answering it lol)

LOL, a majority of my school grade knows this stuff. I guess after the SC everyone tends to work a little ahead.

 

[bleakarcher]

u must go to a gun school if so lol

 

[bleakarcher]

lol

 

[Pfortune35]

solution to roation around x axis given above is correct

for y axis

having tech diffuculties

 

[polpe]

the fuck, cant get the mathematical notation shit anymore

 

[nazfiz]

hey guys, I've got another question. sorry to be a pain.

A drinking glass has the shape of a truncated cone. If the internal radii of the base and the top are 3cm and 4 cm respectively and the depth is 10cm, find by integration, its capacity. If the glass is filled with water to a depth of 5cm, find the volume of the water.

 

[bleakarcher]

dude, im pretty sure that is 4 unit maths, but i did it anywayz. i got 127pi/3 cm^3 for the volume, hence the capacity is 127pi/3 mL. where did this question come from?

 

[Alkanes]

Lol that's not 4U just looks like 4U. That question is in the fitzpatrick 2U textbook, i remember it cos i didn't get it!

 

[bleakarcher]

i used 4U lol

 

[sick_kent]

hey guys, I've got another question. sorry to be a pain.

A drinking glass has the shape of a truncated cone. If the internal radii of the base and the top are 3cm and 4 cm respectively and the depth is 10cm, find by integration, its capacity. If the glass is filled with water to a depth of 5cm, find the volume of the water.

To do it with Integration;

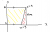
Would you not Intergrate;(Yellow Area+Red Area being A1, Red Area being A2)

Sorry, fucked up integration bit,

pi (integrand) from 1 to 10 (x=10)^2 -((y - 30)/10 = x)^2

 

[sick_kent]

Then the same for part b with the volume of water

PS. sorry for the poor diagram above, my paint handiwork is not of the highest standards

 

[bleakarcher]

^ i lold at this attempt