Loci question I haven't encountered a lot (1 Viewer)

[Run hard@thehsc]

How would you do this question - I haven't really done any locus questions with ellipses. Thanks

 

[5uckerberg]

View attachment 34404
How would you do this question - I haven't really done any locus questions with ellipses. Thanks

The Centre of the ellipse is the midpoint of
part ii Sketch the ellipse taking into account of the equation from the question then for the lengths of axis they are looking for how long the lengths is on the x and y axis. Just think of how far you have stretched the dough
part iii Find the lowest point which should be found from the vertical lengths and the highest point and you are done.
part iii Find the

 

[CM_Tutor]

This question really relates to the old syllabus where ellipses were studied as part of the conics module. It really isn't reasonable to ask without the coverage of conics.

 

[CM_Tutor]

One definition of an ellipse that is mathematically equivalent to the focus-directrix definition is:

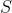
An ellipse is the locus of points that are related to two distinct fixed points and (its foci) by the relationship

where ​

from which it follows that:

• the centre of the ellipse is at the midpoint of
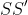
• the fixed points 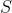and lie on the major axis, which has length 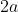between the intercepts
• the ellipse is symmetrical (ie. each has is a mirror image) in the major and minor axis, the minor axis being perpendicular to the major axis and through the centre.

Note that:

• The requirement that 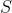and be distinct is because when they coincide, the locus becomes a circle.
• If 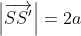then the locus is a single point, the midpoint of .
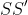• If 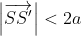then there is no locus.

Using vectors, these facts should be sufficient to solve the rest of the problem.

Consequently, a question like this could be asked as an example of the overlap between the complex numbers and vectors topics, so long as sufficient support material was provided.