Integration is hard - unlike differentiation it's not a mechanical process: there isn't a set sequence of steps you can take that always gives you the answer. In fact, most functions don't even have antiderivatives.
The best way to get better is to just simply practice. You'll develop an intuition for what techniques are more likely to work for a certain integral.
In MX2, you'll mainly be using the following:
-
-substitution
This method can be deceptively tricky, but in the scope of MX2 you shouldn't have many problems with it.
Usually the substitution is not hard to find. For example, if you see a certain function appear several times in the integral then you might try that function as a substitution.
There are two types of substitutions you can try: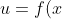
)
, or
)
.
The main difference with these two is what happens with the differential
.
For the former, you'll have to find some function of
to take out of the integrand and combine with
to turn into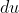
, e.g.
^2(\sin^4x)\, (\cos x \,dx) \stackrel{u\, = \, \sin x}{=} \int (1-u^2)u^4 \, du)
For the latter you don't have to do this - the
will expand into a function of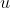
multiplied by
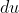. This can give you more to work with.
e.g.
)
-
Integration by parts
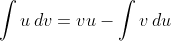
In general you want to use this on integrands that are a product of two functions - one should be easy to differentiate, and the other should be easy to integrate. Ideally these functions should not become more "complicated" after differentiation/integration.
Some examples are
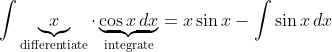
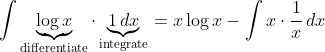
In some cases, the latter function could simply be the constant function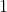
. This is quite useful for many functions, including all the inverse trig ones.
-
Partial fraction decomposition
This is for integrating rational functions, where the degree of the denominator is higher than that of the numerator, and the denominator can be factorised. It's fairly straightforward, but one thing to remember is that if you have a repeated power of a factor then your decomposition needs to contain every decreasing power of that factor, e.g.
^3} = \frac{A}{x-1} + \frac{B}{(x-1)^2} + \frac{C}{(x-1)^3} )
As well as these, you can often algebraically manipulate the integrand into a form where the integration method becomes easy to see, especially if you're dealing with trig functions. So before you even start trying to integrate, see if you can play around with the integrand to make things easier.
For example, completing the square can be helpful.
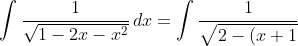^2}}\, dx=\sin^{-1}\left(\frac{x+1}{\sqrt 2}\right)+C)
) ) as it is just so interesting and satisfying when you get it right. That is why I would like to know some tips on integration, more specifically how to identify the correct method and what are all of the methods used in 4u HSC. Thank you so much in advance.
