4 Unit Revising Marathon HSC '10 (2 Viewers)

[jet]

OK, now that 2010 is here I am reviving the old Revision Marathon game.

Rules:
The OP posts a question, first person to get it correct asks the next question, and so on.
Full working MUST be shown before the answer is deemed correct.
Please try to write your solutions in LaTeX. If you can't, I will write it in LaTeX for you after you have posted it.

First Question


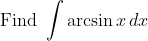
For those of you out of the loop,

 

[xXmuffin0manXx]

=

 

[xXmuffin0manXx]

btw jetblack..ill think ill get u to teach me conics..ill talk to you on msn

i wont be on till later..im gonig to matrix now ill talk to you then

 

[addikaye03]

int. arcsinx dx

By parts (where u=arcsinx)

=(xarcsinx)-int x/sqrt(1-x^2) [this can be solved by letting x=sin@, amongst other ways like noting d/dx [(1-x^2)^-1/2] gives you that]

=(xarcsinx)-rt(1-x^2)+C

{really have to start using LAtex}

 

[jet]

=

Do you have full working for me? Or is wolfram alpha good enough?

int. arcsinx dx

By parts (where u=arcsinx)

=(xarcsinx)-int x/sqrt(1-x^2) [this can be solved by letting x=sin@, amongst other ways like noting d/dx [(1-x^2)^-1/2] gives you that]

=(xarcsinx)-rt(1-x^2)+C

{really have to start using LAtex}

I wrote a guide for you guys. It's stickied in all forums but general maths.

 

[xXmuffin0manXx]

Do you have full working for me? Or is wolfram alpha good enough?



I wrote a guide for you guys. It's stickied in all forums but general maths.

got it from matrix book hahaha..which coincidentally..the working is written out in my handwriting

 

[addikaye03]

btw jetblack..ill think ill get u to teach me conics..ill talk to you on msn

i wont be on till later..im gonig to matrix now ill talk to you then

Shit Q imo, just tedious:

z=cos@+isin@, z^-1=cos(@)-isin(@) {using demoivres}

So z-z^-1=cos@+isin@-(cos@+isin@)=> 2isin@

(z-z^-1)^6=(2isin@)^6

64i^6sin^6@=6C0(z^6)+6C1(z^5)(-z)^1+6C2(z^4)(-z^-1)^2+6C3(z^3)(-z^-1)^3...+6C6(-z^-1)^6

-64sin^6@= Simplifed RHS

Then you get the expression.

Question: Solve (z-1)^6+(z+1)^6=0

 

[shaon0]

Shit Q imo, just tedious:

z=cos@+isin@, z^-1=cos(@)-isin(@) {using demoivres}

So z-z^-1=cos@+isin@-(cos@+isin@)=> 2isin@

(z-z^-1)^6=(2isin@)^6

64i^6sin^6@=6C0(z^6)+6C1(z^5)(-z)^1+6C2(z^4)(-z^-1)^2+6C3(z^3)(-z^-1)^3...+6C6(-z^-1)^6

-64sin^6@= Simplifed RHS

Then you get the expression.

Question: Solve (z-1)^6+(z+1)^6=0

Cancel every odd term:
(z-1)^6+(z+1)^6=0
2(z^6+15z^4+15z^2+1)=0
2(z^2+1)(z^4+14z^2+1)=0
Thus, z=+-i OR
z^2=(-14+-sqrt(14^2-4))/2
z^2=-(7+-4sqrt(3))
Thus, z=+-isqrt(7+-4sqrt(3))

 

[addikaye03]

Cancel every odd term:
(z-1)^6+(z+1)^6=0
2(z^6+15z^4+15z^2+1)=0
2(z^2+1)(z^4+14z^2+1)=0
Thus, z=+-i OR
z^2=(-14+-sqrt(14^2-4))/2
z^2=-(7+-4sqrt(3))
Thus, z=+-isqrt(7+-4sqrt(3))

I guess that would work, neat solution!! Not how i did it.

Spoiler

Mine: (1-z)^6+(1+z)^6=0

Dividing by (1+z)^6; [(1-z)/(1+z)]^6=-1

(1-z)/(1+z)=cis(2k+1)/6 where k=0,+-1, +-2, -3

1-z=cis(2k+1)/6+cis(2k+1)/6z

1-cis(2k+1)/6=[cis(2k+1)+1]z

z=(1-cis@)/(1+cis@) where @=(2k+1)/6

Use double angles to simplify expression, sub in values of k

Question: Let w be a zero of the polynomial P(z)=4z^8-17z^4+4

a)Show that iw and w^-1 are also the roots of P(z)=0

b)Hence, find all the roots of P(z)=0

 

[shaon0]

I guess that would work, neat solution!! Not how i did it.

Spoiler

Mine: (1-z)^6+(1+z)^6=0

Dividing by (1+z)^6; [(1-z)/(1+z)]^6=-1

(1-z)/(1+z)=cis(2k+1)/6 where k=0,+-1, +-2, -3

1-z=cis(2k+1)/6+cis(2k+1)/6z

1-cis(2k+1)/6=[cis(2k+1)+1]z

z=(1-cis@)/(1+cis@) where @=(2k+1)/6

Use double angles to simplify expression, sub in values of k

Question: Let w be a zero of the polynomial P(z)=4z^8-17z^4+4

a)Show that iw and w^-1 are also the roots of P(z)=0

b)Hence, find all the roots of P(z)=0

a)
P(iw)=4w^8-17w^4+4=P(w)
As w is a root of P(z) ie P(w)=0. It follows P(iw)=0
P(1/w)=4(1/w^8)-17/w^4+4
=[4w^8-17w^4+4]/w^8
=P(w)/w^8
=0

b)
Let y=w^4:
P(y)=4y^2-17y+4
y=[+17+-sqrt(17^2-64)]/8
y=4or 1/4

w=+-1/sqrt(2), +-sqrt(2), +-isqrt(2), +-i/sqrt(2)

 

[Trebla]

Here's a new question and a big LOL at it haha!

 

[kaz1]

Who said Maths and English can't be friends? lol

 

[study-freak]

lolllll

 

[cutemouse]

That is from Cambridge!

Oh wait no... I recall seeing it in my friend's First year lecture notes for USyd for some calculus topic...

 

[Trebla]

Oh wait no... I recall seeing it in my friend's First year lecture notes for USyd for some calculus topic...

You'd be right. Makes maths seem a bit more interesting don't you think? lol

Anyway, is anyone going to solve it?

 

[xXmuffin0manXx]

fkn lol..

please someone solve it

 

[jet]

If it's not done by 4pm tomorrow, I'll solve it.

 

[shaon0]

You'd be right. Makes maths seem a bit more interesting don't you think? lol

Anyway, is anyone going to solve it?

I've solved the question but idk if it's a correct method as i haven't learned 2nd ODE properly. Thus i won't be posting a "solution."

 

[untouchablecuz]

not sure about their fate, tho i do know that - their love forms an ellipse

 

[shaon0]

I got something else for d) but i'm probably wrong. Can someone post up another question? Preferably not uni maths.
EDIT: Yeah, i'm wrong about d)