HSC 2013 MX2 Marathon (archive) (7 Viewers)

nightweaver066 · Jan 14, 2013

Re: HSC 2013 4U Marathon

Trebla said:
There is a contradiction in your working.

I don't think it's a contradiction, i just made a mistake in the 5th line so i technically didn't prove the left side of the inequality

Sy123 · Jan 14, 2013

Re: HSC 2013 4U Marathon

asianese said:
Isn't it $\lim_{n\to\infty} \left(1+\frac{1}{n}\right)^n = e$ not the other way around? idk O_O

The 'proof' that

$e \rightarrow \sum_{j=0}^{\infty} \frac{1}{j!} \text{ if } n\rightarrow \infty$ doesn't make sense either - e is just a constant, it shouldn't matter what n is doing! (I think it's a directional issue here)

Apologies, it should be the other way around. Fixed now.

Sy123 · Jan 15, 2013

Re: HSC 2013 4U Marathon

$A circle of unit radius is rolled along the x-axis in the positive direction initially at the origin. There is a red marker on circle that is fixed on the circle as it rolls along the x-axis. The marker is originally at the origin, and as the ball moves, the marker begins to move in a clock-wise direction. The ball makes 1 revolution in 1 second.$

$Show that the cartesian equation of the red marker is$

$x=\cos^{-1}(1-y)-\sqrt{y(2-y)}$

$It looks like this$

twinklegal19 · Jan 15, 2013

y=|sinx|?

Edit: dunno if that's the right motion equation since I'm not sure how to incorporate the fact that 1 revolution = 1 second

Sy123 · Jan 15, 2013

Re: HSC 2013 4U Marathon

twinklegal19 said:
y=|sinx|?

Edit: dunno if that's the right motion equation since I'm not sure how to incorporate the fact that 1 revolution = 1 second

Not quite heh, I made it a show that to compare solutions

twinklegal19 · Jan 15, 2013

Re: HSC 2013 4U Marathon

Sy123 said:
Not quite heh, I made it a show that to compare solutions

ahaha

yeah, I got the curve equation out by inspection...

if only it was that simple!

Sy123 · Jan 15, 2013

Re: HSC 2013 4U Marathon

twinklegal19 said:
ahaha

yeah, I got the curve equation out by inspection...

if only it was that simple!

HINT: Parametric equations

RealiseNothing · Jan 15, 2013

Re: HSC 2013 4U Marathon

twinklegal19 said:
y=|sinx|?

Edit: dunno if that's the right motion equation since I'm not sure how to incorporate the fact that 1 revolution = 1 second

I'm pretty sure the speed the circle turns is irrelevant as it would still trace the same path.

RealiseNothing · Jan 15, 2013

Re: HSC 2013 4U Marathon

twinklegal19 said:
ahaha

yeah, I got the curve equation out by inspection...

if only it was that simple!

.

Sy123 · Jan 15, 2013

Re: HSC 2013 4U Marathon

RealiseNothing said:
I'm pretty sure the speed the circle turns is irrelevant as it would still trace the same path.

This is true, I gave it to sort of make the elimination of variables easier. Because its easier to imagine it if you are given a time

But reason why this is true is:

$l=r \theta \rightarrow l=\theta$

barbernator · Jan 15, 2013

Re: HSC 2013 4U Marathon

good question sy

Sy123 · Jan 15, 2013

Re: HSC 2013 4U Marathon

Yeah, my answer was wrong since I messed up the initial conditions, it should be correct now.

barbernator · Jan 15, 2013

Re: HSC 2013 4U Marathon

Sy123 said:
Yeah, my answer was wrong since I messed up the initial conditions, it should be correct now.

thought so lol, ill post sol tomorrow if nobody does

Sy123 · Jan 15, 2013

Re: HSC 2013 4U Marathon

$$Prove that if$ \ \ \cos n\theta \ \ \ $would be expressed in terms of$ \ \ \cos \theta \ \ $ then $ \ \ \cos n\theta \ \ $would be a polynomial of degree n in$ \ \ \cos \theta$

Sy123 · Jan 16, 2013

Re: HSC 2013 4U Marathon

Sy123 said:
$A circle of unit radius is rolled along the x-axis in the positive direction initially at the origin. There is a red marker on circle that is fixed on the circle as it rolls along the x-axis. The marker is originally at the origin, and as the ball moves, the marker begins to move in a clock-wise direction. The ball makes 1 revolution in 1 second.$

$Show that the cartesian equation of the red marker is$

$x=\cos^{-1}(1-y)-\sqrt{y(2-y)}$

$It looks like this$

Here is my solution to the question.

==============

Ok, so we need to model the moving marker, and I know I can simplify the problem by finding the parametric equations of the red marker.
So I need to find the parametric equations of the marker, but since this red marker is dependent on the circle. I will first investigate the circle to see what I can find:

Establish the equation of the circle:

$(x-p)^2+(y-1)^2 = 1$

For some variable centre (p,1).

Now I know that the parametric equations of this circle is:

$x=p+\cos \theta$
$y=1+\sin \theta$

These equations are able to give me any point I want on that circle, and the point we want to model or represent is the red marker.
So we need to find a relationship between p and theta such that they model the red marker.

This is why the time variable is useful to imagine the following situation. Ok, so our circle makes 1 revolution per second, but its rolling, hence the distance the centre covers in 1 second, is equal to the circumference (draw this out if it doesn't make sense)

Hence, $p=2\pi t$
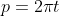
Now we need to find a way to model theta in terms of t. This is easy enough anyway, every second theta increases by 2pi, hence

$\theta = 2\pi t$

However we need to be careful here, theta is actually decreasing, as in it is increasing in the negative direction since our theta is going clockwise (as stated in the question), hence indeed it comes about that:

$\theta = -2\pi t$

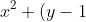Recall the initial condition for the red marker being (0,0) When the circle has an equation of $x^2+(y-1)^2 = 1$
However when theta is zero initially, our inital conditions become (1,1). To make our inital conditions (0,0) we must take away pi/2 to theta (imagine the unit circle of trigonometry, similar logic is involved).

Hence our new equations are:

$x=2\pi t + \cos (-2\pi t - \frac{\pi}{2})$

$y= 1+ \sin (-2\pi t - \frac{\pi }{2} )$

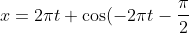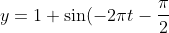Simplification

$x=2\pi t - \sin (2\pi t)$

$y=1- \cos (2\pi t)$

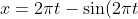Now we have the parametric equations, we now need to isolate one and form the cartesian equation:

$2\pi t = \cos^{-1} (1-y)$

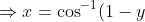$\Rightarrow x= \cos^{-1}(1-y) - \sin (\cos^{-1}(1-y))$

Use the right angle triangle in order to simplify the second term

$\therefore \ x=\cos^{-1}(1-y) - \sqrt{y(2-y)}$

Sy123 · Jan 18, 2013

Re: HSC 2013 4U Marathon

Here is a nice result I proved:

$$Consider the polynomial$ \ \ \ \ P(z) = z^n + \sum_{k=0}^{n-1} b_{k}z^k$

$$Where$ \ \ \ b_k \in \mathbb{R} \ \ \ $for$ \ \ k=0,1,2,...(n-1)$

$$Prove that if ALL roots are real then$ \ \ \ \ \ b_{n-1}^2 > 2b_{n-2}$

vbzxwgy · Jan 19, 2013

Re: HSC 2013 4U Marathon

Sy123 said:
$$Hence show that if$ \ \ \ \ b_k < 0 \ \ \ $for$ \ \ k=0,1,2,...(n-1)$

$Then ALL roots are real$

not true.

RealiseNothing · Jan 19, 2013

Re: HSC 2013 4U Marathon

Sy123 said:
Here is a nice result I proved:

$$Consider the polynomial$ \ \ \ \ P(z) = z^n + \sum_{k=0}^{n-1} b_{k}z^k$

$$Where$ \ \ \ b_k \in \mathbb{R} \ \ \ $for$ \ \ k=0,1,2,...(n-1)$

$$Prove that if ALL roots are real then$ \ \ \ \ \ b_{n-1}^2 > 2b_{n-2}$

$$Hence show that if$ \ \ \ \ b_k < 0 \ \ \ $for$ \ \ k=0,1,2,...(n-1)$

$Then ALL roots are real$

It only works one way. If all roots are real, then $b_{n-1}^2 > 2b_{n-2}$ . However if $b_{n-1}^2 > 2b_{n-2}$ , then it doesn't mean all roots are real.

Sy123 · Jan 19, 2013

Re: HSC 2013 4U Marathon

vbzxwgy said:
not true.

RealiseNothing said:
It only works one way. If all roots are real, then $b_{n-1}^2 > 2b_{n-2}$ . However if $b_{n-1}^2 > 2b_{n-2}$ , then it doesn't mean all roots are real.

Very true, ignore the last part then.

Sy123 · Jan 20, 2013

Re: HSC 2013 4U Marathon

A neat result that I proved:

$Functions$ \ \ \ g(x) \ \ $and$ \ \ h(x) $are said to be within the same family if$ \ \ g(x) = k h(x) \ \ $ for some constant k value$

$i.e.$ \ \ \ g(x) = 2e^x \ \ \ \ h(x)=5e^x \ \ \ $are within the same family of functions$

$$Consider the sequence of functions$ \ \ \ f_1 , f_2, f_3 , .... , f_n$

$$Each consecutive function is constructed via finding the tangent to the variable point$ \ \ \ P(t, f(t))$

$$This tangent then intersects the x-axis at$ \ \ Q(g(t), 0 ) \ \ $and intersects the y-axis at$ \ \ R(0, h(t))$

$$It then follows that the consecutive function is the locus of the variable point$ \ \ \ S(g(t), h(t))$

$$i) Given that$ \ \ \ f_1 = (t, f(t))$

$$Show that$ \ \ \ f_2 = (t - \frac{f(t)}{f'(t)} , f(t) - t f'(t) ) \ \ \ \ \fbox{2}$

$$ii) Consider functions$ \ \ \ A = (\alpha, A(\alpha)) \ \ \ \ B = (k\alpha, C \cdot A(\alpha)) \ \ \ \ $(for some constant value of k and C). Find all families of functions whereby A and B are within the same family. (within the HSC syllabus)$ \ \ \ \ \fbox {3}$

$$iii) Show that if$ \ \ \ f_1, f_2, f_3, .... , f_n \ \ \ $ are ALL within the same family of functions, then$ \ \ \ f_1 \ \ \ $ must satisfy$ \\ \\ f_1 = kx^n \\ \\ $for some constant k and number n$ \ \ \ \ \ \ \fbox{2}$

HSC 2013 MX2 Marathon (archive) (7 Viewers)

Well-Known Member

This too shall pass

This too shall pass

.

This too shall pass

.

This too shall pass

what is that?It is Cowpea

what is that?It is Cowpea

This too shall pass

Active Member

This too shall pass

Active Member

This too shall pass

This too shall pass

This too shall pass

New Member

what is that?It is Cowpea

This too shall pass

This too shall pass

Users Who Are Viewing This Thread (Users: 0, Guests: 7)