-
Looking for HSC notes and resources? Check out our Notes & Resources page
Make a Difference – Donate to Bored of Studies!
Students helping students, join us in improving Bored of Studies by donating and supporting future students!
MX2 Integration Marathon (3 Viewers)
- Thread starter dan964
- Start date
stupid_girl
Active Member
- Joined
- Dec 6, 2009
- Messages
- 221
- Gender
- Undisclosed
- HSC
- N/A
Yes, it is a good idea (unless you want to use trig identities many times or prove a reduction formula first)Let t=tanx is a good idea?
stupid_girl
Active Member
- Joined
- Dec 6, 2009
- Messages
- 221
- Gender
- Undisclosed
- HSC
- N/A
The algebra is quite annoying.
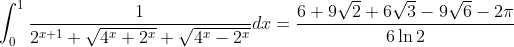
stupid_girl
Active Member
- Joined
- Dec 6, 2009
- Messages
- 221
- Gender
- Undisclosed
- HSC
- N/A
I guess you must have got an answer close to zero.Let t=tanx is a good idea?
ratman1242
New Member
- Joined
- Sep 29, 2019
- Messages
- 1
- Gender
- Undisclosed
- HSC
- 2019
This one is fun
 \ln ( \tan (x)) dx)
stupid_girl
Active Member
- Joined
- Dec 6, 2009
- Messages
- 221
- Gender
- Undisclosed
- HSC
- N/A
With the usual trick, f(x)+f(pi/2-x)=-cos(2x) ln(tan x), which can be evaluated by IBP.This one is fun
 \ln ( \tan (x)) dx)
stupid_girl
Active Member
- Joined
- Dec 6, 2009
- Messages
- 221
- Gender
- Undisclosed
- HSC
- N/A
The following can be solved similarly.
Last edited:
stupid_girl
Active Member
- Joined
- Dec 6, 2009
- Messages
- 221
- Gender
- Undisclosed
- HSC
- N/A
This one is absolutely painful.
^{2}}{x+e^{x}+\sqrt{e^{3x}+xe^{2x}}}\right)dx)
-2+2\sqrt{e+1}-\ln4)
TheOnePheeph
Active Member
- Joined
- Dec 13, 2018
- Messages
- 241
- Gender
- Male
- HSC
- 2019
HSC is over but a good integral is always fun:This one is absolutely painful.
-2+2\sqrt{e+1}-\ln4)
Last edited:
stupid_girl
Active Member
- Joined
- Dec 6, 2009
- Messages
- 221
- Gender
- Undisclosed
- HSC
- N/A
I have skipped the steps of rationalization.The algebra is quite annoying.
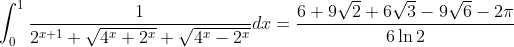
stupid_girl
Active Member
- Joined
- Dec 6, 2009
- Messages
- 221
- Gender
- Undisclosed
- HSC
- N/A
A new one
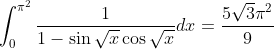
Paradoxica
-insert title here-
Hey you can't just commute the limit with the integral like that. Everyone knows limits don't commute in general.Taking limit

Please justify the commutation using only techniques from the syllabus.
stupid_girl
Active Member
- Joined
- Dec 6, 2009
- Messages
- 221
- Gender
- Undisclosed
- HSC
- N/A
It requires uniform convergence. Does the syllabus cover techniques to prove uniform convergence?Hey you can't just commute the limit with the integral like that. Everyone knows limits don't commute in general.
Please justify the commutation using only techniques from the syllabus.
HeroWise
Active Member
- Joined
- Dec 8, 2017
- Messages
- 347
- Gender
- Male
- HSC
- 2020
@stupid_girl teach us
stupid_girl
Active Member
- Joined
- Dec 6, 2009
- Messages
- 221
- Gender
- Undisclosed
- HSC
- N/A
It is too abstract. I'll leave it for your university lecturer and tutor.@stupid_girl teach us
Is that another way of saying “the proof is left as an exercise for the reader”?It is too abstract. I'll leave it for your university lecturer and tutor.
stupid_girl
Active Member
- Joined
- Dec 6, 2009
- Messages
- 221
- Gender
- Undisclosed
- HSC
- N/A
This one isn't too hard, right? The same old trick applies.A new one
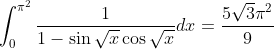
stupid_girl
Active Member
- Joined
- Dec 6, 2009
- Messages
- 221
- Gender
- Undisclosed
- HSC
- N/A
(where k is a real constant)
stupid_girl
Active Member
- Joined
- Dec 6, 2009
- Messages
- 221
- Gender
- Undisclosed
- HSC
- N/A
This one is similar but the algebra is slightly longer.
-\left(k^{2}+1\right)\left(\tan^{-1}k\right)\ln\left(k^{2}+1\right)}{k^{2}\left(k^{2}+1\right)})
